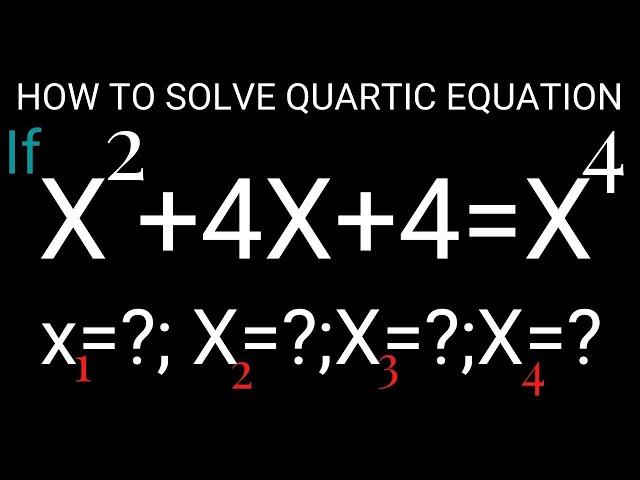
How To Solve Quartic Equation | Solve X^2+4X+4=x^2 | #exponentialequations | #olympiad | Quartic

How To Solve The Quartic Equation X^2+4X+4=x^4 systematically. #exponentialequations #olympiadmathematics

In this video, I will show you the way round on how to solve the above quartic equation by using difference of two square to formulate two separate quadratic equations after rearranging the terms in the equation.

You will learn how to solve the resulted quadratic equations separately by using the formula method.
Also in this video, you be be introduced to how to solve a quadratic equation with the imagery roots by introducing the “i” symbol to replace the negative one.

These and many more tricks about Mathematics, exponential problems, Olympiad mathematics questions, Olympiad exams etc, you will be exposed to in this video and to get all the listed features please kindly watch this video from the beginning to the end without skipping any parts for the details.

Above all, if you are new to this channel, kindly subscribe and turn on the bell notification button to get all new updates as we upload at least one video everyday in this channel.

Thanks for being there !!!

#exponentialequations #mathstricks
#olympiadmathematicscompetition #olympiadpreparation #olympiad #olympiadexams
#exponents #mathtrick
#exponential
#onlinemaths
#onlinemathsclasses
#formulamethod
#differenceofsquares
#zeroproductsproperty

Тэги:

#How_To_Solve_Quartic_Equation #quartic_equations #tricks_to_quartic_equations #difference_of_two_square #application_of_difference_of_ttwo_square #Olympiad_math_tricks #Olympiad_mathematics_questions #secrets_to_quartic_equations #quadratic_equations #formula_method_to_quadratic_equations #application_of_the_imagery_symbol_“i“ #real_roots #imagery_roots #mathematics_tricks #exponential_tricks #factorization_of_power #Olympiad_maths #zero_product_property #exponents

Скачать видео