Комментарии:

Even though she's saying nonsense but i trust her she looks like a 🤓
In a good way though 🤓😊👍😂😉😜😔😞

i try to watch this video but not playing just circle is moving
Ответить
Now I need English version.
Ответить
Beautiful explanation, concise and simple. Thank you
Ответить
Thank you for a good a concise answer to my question! 🤝
Ответить
Simple and get to the point, thank you!
Ответить
Thank you SO much! I only found a lot of mathematical explanations. This little drawing explaining that it's just one vector projected onto another, was all I was looking for.
Ответить
you’re amongst my favorite persons now. Great explanation <3
Ответить
TYSM for the video! literally could not find another answer to my question of what the dot product is "saying" and you had it in literally the first 5 seconds.
Ответить
This is so beautiful I finally get it!!! 😭
Ответить
Very thanks!!!
Ответить
Thank you
Ответить
ty !!!! this helped a lot !
Ответить
This is a really interesting way to conceptually understand the dot product, thanks!
Ответить
Looks awfully similiar to cosine
Ответить
wow wtf i did not expect to understand this in literally 50 seconds LMAO thank you
Ответить
And then cross product to see if A is to the left or right of B. Fancy way of knowing whether your rogue can apply that nasty backstab multiplier or tell your AI driver to steer left or right to avoid stuff in your level! ;)
Ответить
Thank you so much!
Ответить
Keep up the good work!
Ответить
Amazing!
Ответить
just solved my problems in about 45 seconds thank you!
Ответить
Great video! I appreciate how concise this was!
Ответить
NICE!
Im going to use this to confirm if my bots are facing their target's before they can attack within a FoV!

Damn, i have seen hours long videos on dot product! When you know, you know! Thanks Freya..
Ответить
Thank you for your video!!
Ответить
damn, that a pro explanation 1m and I understood perfectly lol
Ответить
i think you meant to say parallel hehehehheheeeeeee
Ответить
wow this explained it really fast
Ответить
This actually makes perfect sense! Now i perfectly understand how dot products work in my code
Ответить
man, whenever im trying to understand some game dev math stuff i really should come to this channel first
Ответить
you are a live saver!
Ответить
@Freya what does "normalized" vector mean that she talks about in her description!?
Ответить
brilliant explanation, thank you so much for the simple illustration
Ответить
Nice
Ответить
Thanks a lot🎉
Ответить
I wish I could tick a "love" emoji instead of just like. Most concise explanation I've found so far.
Ответить
Wow this explanation is crazy intuitive, thank you so much
Ответить
What about Z axis?
Ответить
made my day. happy to find your channel
Ответить
Such a good explanation
Ответить
Only if the module of both vectors are 1... Cuz dot product is a product of 'the parallel part of b in respect to a' and a
Ответить
Very helpful video!
Ответить
now I understand how the hessian normal form works (you only normalize the normal vector in that one), thank you miss Freya!
Ответить
Very helpful, thanks a lot
Ответить
Am a senior engineering student and never quite thought about it this way. Props!
Ответить
my fix was to normalize them...
Ответить
I'm sorry if this come off as a dumb question, i'm not really good with math,
For this to work rightly the 2 vectors must stem from the same origin right ?

This is not quite accurate. In reality, u⋅v = magnitude of the projection of u unto v times the magnitude of v. she said that it is simply the magnitude of the projection of u unto v.
Ответить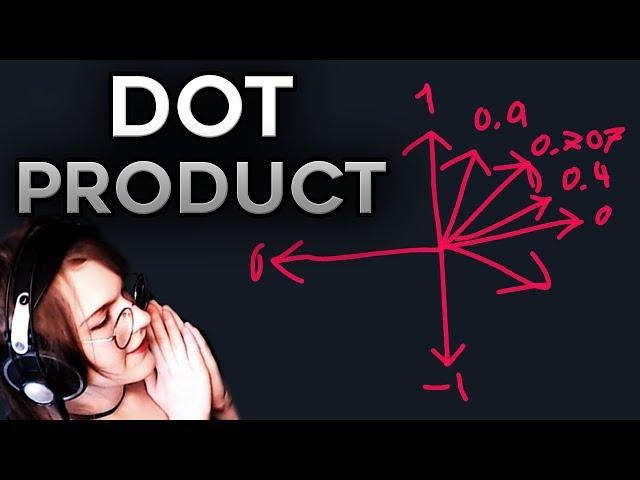


![Скрижаль Исет [The Tablet Of Iset] Скрижаль Исет [The Tablet Of Iset]](https://invideo.cc/img/upload/QUZUNmtUZVdHMlA.jpg)